HP Prime for All

Articles : Exploring Quadrilaterals

In this activity, we use the Geometry app to create a quadrilateral. We then create and connect the midpoints of consecutive sides of the quadrilateral to form another quadrilateral and explore the properties of the latter in terms of the former.

1. Press I and tap the Geometry icon. The app opens in its Plot view.
2. Tap Cmds, select 4 Polygon > 4 Quadrilateral.
3. Tap a location and press E to select the first vertex of the quadrilateral. Continue to tap and press E to select the other three vertices. press J to deselect the quadrilateral command.
   The display now shows a quadrilateral named E, based on the four points A, B, C, and D.
4. Tap Cmds and select 2 Point > 3 Midpoint . Tap near the midpoint of AB (or tap each of points separately) and press E. Continue likewise to create the midpoints of the other segments. When you are done, press J to deselect the midpoint command.
5. Repeat Steps 2 and 3 to create quadrilateral O from points K, L, M, and N.
   The display now shows both quadrilaterals. We will now tidy up our construction and making it colorful before beginning our explorations.
6. Press Y to display the Symbolic view of the app. Here, each of the geometric objects is defined symbolically. Objects with checks besides them are displayed in the Plot view. Uncheck each segment by highlighting it and pressing ✓. Scroll down to uncheck the segments KL, LM, MN, and NK as well.
7. With the segments hidden, return to the Plot view (press P ) and give the quadrilaterals their own colors. Touch outer quadrilateral and tap Options > 1 Choose Color (or Z > 4 Choose Color) and select any color from color picker.
8. Repeat Step 7 to select dark blue for the inner quadrilateral.
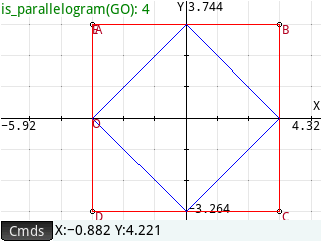
9. Press M to open the Numeric view of the app. Here we define measurements and tests involving our geometric objects. Tap New to start a new measurement. In this case, it is a test. Tap Cmds to open the menu of commands select A Tests, then select 8 Parallelogram . The command is pasted into the command line. Remember that the name of our inner quadrilateral is GO. Type GO between the parentheses and press E. Tap ✓ to check this test for display in the Plot view.
10. Return to the Plot view to see our constructions and the test result. Drag anywhere outside of the construction to pan the plane so you can see both the test result and the construction.

We are now ready to explore our construction.

1. Select one of the vertices of the outer quadrilateral by tapping on it and pressing E . You can now drag it anywhere within the display with your finger. As you move a vertex, notice that the parallelogram test on KLMN maintains a value of 1, indicating it is always a parallelogram. The parallelogram test can return any of 5 values:
   
   • 0. Not a parallelogram
   • 1. A parallelogram only
   • 2. A rhombus
   • 3. A rectangle
   • 4. A square
2. Press Y to return to the Symbolic view. Here you can give the coordinates of points A, B, C, and D exact values.
3. Select GA, tap and enter new coordinates (-3,3). Tap when you are done.
4. Repeat Step 3 with points B, C, and D so that you have A(-3,3), B(3,3), C(3,-3) and D(-3,-3), making ABCD a square.
5. Press P to return to the Plot view. The display shows the parallelogram test now has a value of 4, indicating that KLMN is a square as well. It seems that KLMN is always at least a parallelogram, no matter where we move the coordinates of points A, B, C, and D (as long as they are not collinear!). To see why this is so, simply construct the diagonals AC and BD.
6. Tap Cmds and select 3 Line > 1 Segment. Tap on point A and press E. Then tap on point C and press E . Repeat with points B and D. Then press J to deselect the command. Since segment KL joins the midpoints of two sides of ΔABC, then it is parallel to the third side AC; likewise, MN joins the midpoints of two sides of ΔACD, and is thus parallel to AC as well. Thus KL and MN are parallel. The same can be seen for segments NK and ML.
7. Press I and tap to save your construction with a name you’ll remember.

With the testing and symbolic abilities of the Geometry app, students can set about the proofs or refutations of conjectures such as these:

• If ABCD is a rhombus, then KLMN is a rectangle
• If ABCD is a rectangle, then KLMN is a rhombus
• If ABCD is an isosceles trapezoid, then KLMN is a rhombus
• If the diagonals of ABCD are perpendicular, then KLMN is a rectangle
• If the diagonals of ABCD are congruent, then KLMN is a rhombus

Comments